Determine the number of atoms, N, in the given amount of substance. We do this by multiplying the number of moles, n, by Avogadro's number, A,. See full answer below. The number of atoms in a weighed sample is related to electron flow to calculate Avogadro's number. In this electrolytic cell, both electrodes are copper and the electrolyte is 0.5 M H 2 SO 4. During electrolysis, the copper electrode ( anode ) connected to the positive pin of the power supply loses mass as the copper atoms are converted to.
Chemistry- Avogadro's number, 6.022. 10 23 is the number of things in one mole. The question indicates that there is 1 mole of H 2. Thus there are 6.022. 10 23 molecules of H 2. However the question is asking for the amount of atoms in 1 mole of H 2.
- Avogadro's number, 6.022. 10 23 is the number of things in one mole. The question indicates that there is 1 mole of H 2. Thus there are 6.022. 10 23 molecules of H 2. However the question is asking for the amount of atoms in 1 mole of H 2.
- The Avogadro constant or (the Avogadro number earlier) is the number of elementary units in one mole of any substance. The Avogadro constant is denoted as NA. It has the dimension of the reciprocal amount of substance (mol −1). The approximate value of NA is 6.022 × 10 23 mol −1.
The Avogadro's number is a constant used in analytical chemistry to quantify the number of particles or microscopic entities from macroscopic measurements such as mass. It is very important to know this number in order to understand molecule composition, interactions and combinations. For example, to create a water molecule it is necessary to combine two hydrogen atoms and one oxygen atom to obtain one mole of water. The number of Avogadro is a constant that must be multiplied by the number of atoms of each element to obtain the value of oxygen (6.023 x 1023 atoms of O) and Hydrogen (2x 6.022x 1023) that form a mole of H2O.
What is the Avogadro's number?
The Avogadro's number is a constant that represents the number of existing atoms in twelve grams of 12-pure carbon. This figure makes possible to count microscopic entities. This includes the number of elementary entities (i.e. atoms, electrons, ions, molecules) that exist in a mole of any substance. The Avogadro's number is equal to (6,022 x 10 raised to 23 particles) and is symbolized in the formulas with the letters L or NA. In addition, it is used to make conversions between grams and atomic mass unit. The unit of measure of the Avogadro's number is the mole (mol-1) but it can also be defined in lb/mol-1 and oz/mol-1.
What is the Avogadro’s number?
What Is The Value Of Avogadro's Number
The Avogadro’s number is 602,000,000,000,000,000,000,000,000 which is equal to 602,000 trillion = 6.02 x 1023. This value is found from the number of carbon atoms contained in 12 grams of carbon 12 elevated to power 23.
It is important to mention that depending on the unit of measurement used, the number may vary. In this sense, if you work with mole the number is 6.022140857 (74) x 1023 mole-1.
- If you work with pounds it will be 2.731 597 34(12) × 1026 (Lb.-mol)-1.
- If you work with ounces it will be 1.707 248434 (77) x 1025 (oz-mol)-1.
What does the Avogadro’s number represent?
The Avogadro’s number represents the number of atoms that exist in twelve grams of carbon-12.
This number represents a quantity without an associated physical dimension, so it is considered a pure number to describe a physical characteristic without dimension or explicit unit of expression. For this reason, it has the numerical value of constant that the units of measurement have. Descargar nfs most wanted pc portable.
How the Avogadro’s number is calculated
The Avogadro’s number can be calculated by measuring the Faraday constant (F) which represents the electrical charge carried by a mole of electrons and dividing it by the elementary charge (e). This formula is Na= F/e.
The Avogadro constant can be calculated using analytical chemistry techniques known as coulometry, which determine the amount of matter transformed during the electrolysis reaction by measuring the amount consumed or produced in coulombs.
There are also other methods to calculate it such as the electron mass method, known as CODATA or the system of measuring through crystal density using X-rays.
History
The Avogadro’s number or Avogadro constant is named after the Italian scientist Amedeo Avogadro who in 1811 determined that the volume of a gas at a given pressure and temperature is proportional to the number of atoms or molecules regardless of the nature of the gas.
In 1909, Jean Perrin, a French physicist – winner of the Nobel Prize in physics in 1926 – proposed naming the constant in honor of Avogadro. Perrin, using several methods, proved the use of the Avogadro constant and its validity in many of his works.
Initially, it was called Avogadro’s number to refer to the number of molecules-grams of oxygen but in 1865, the scientist JohannJosef Loschmidf called the Avogadro’s number, Avogadro constant. Loschmidf estimated the average diameter of air molecules by a method equivalent to calculating the number of particles in a specific gas volume. For this reason, the particle density value of an ideal gas is known as the Loschmidt constant, which is approximately proportional to the Avogadro constant. From then on, the symbol for the Avogadro’s number or Avogadro constant can be NA (Avogadro’s number) or L (in honor of Loschmid).
A curious fact in Avogadro’s number history is that the Italian scientist Amedeo Avogadro never measured the volume of any particle in his lifetime because in his time there were no elements necessary to do so, but it is thanks to his contributions that Perrin developed this constant and therefore gave it that name.
Avogadro's Law:
Ten Examples
| Boyle's Law | Combined Gas Law |
| Charles' Law | Ideal Gas Law |
| Gay-Lussac's Law | Dalton's Law |
| Diver's Law | Graham's Law |
| No Name Law | Return to KMT & Gas Laws Menu |
Discovered by Amedo Avogadro, of Avogadro's Hypothesis fame. The ChemTeam is not sure when, but probably sometime in the early 1800s.
Gives the relationship between volume and amount when pressure and temperature are held constant. Remember amount is measured in moles. Also, since volume is one of the variables, that means the container holding the gas is flexible in some way and can expand or contract.
If the amount of gas in a container is increased, the volume increases.
If the amount of gas in a container is decreased, the volume decreases.
Why?
Suppose the amount is increased. This means there are more gas molecules and this will increase the number of impacts on the container walls. This means the gas pressure inside the container will increase (for an instant), becoming greater than the pressure on the outside of the walls. This causes the walls to move outward. Since there is more wall space the impacts will lessen and the pressure will return to its original value.
The mathematical form of Avogadro's Law is:
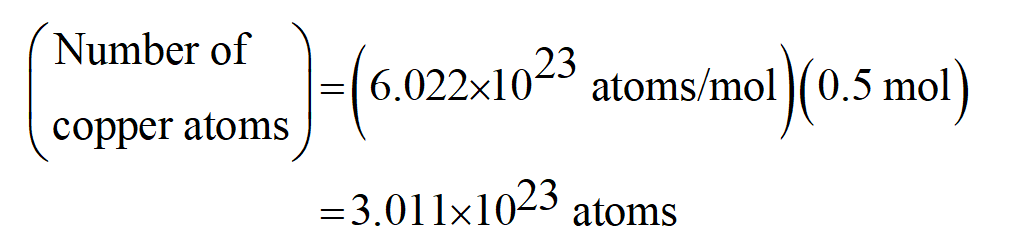
| V | |
| ––– | = k |
| n |
This means that the volume-amount fraction will always generate a constant if the pressure and temperature remain constant.
Let V1 and n1 be a volume-amount pair of data at the start of an experiment. If the amount is changed to a new value called n2, then the volume will change to V2.
We know this:
| V1 | |
| ––– | = k |
| n1 |
And we know this:
| V2 | |
| ––– | = k |
| n2 |
Since k = k, we can conclude:
| V1 | V2 | |
| ––– | = | ––– |
| n1 | n2 |
This equation will be very helpful in solving Avogadro's Law problems. You will also see it rendered thusly:
V1 / n1 = V2 / n2
Sometimes, you will see Avogadro's Law in cross-multiplied form:
V1n2 = V2n1
Avogadro's Law is a direct mathematical relationship. If one gas variable (V or n) changes in value (either up or down), the other variable will also change in the same direction. The constant K will remain the same value.
Example #1: 5.00 L of a gas is known to contain 0.965 mol. If the amount of gas is increased to 1.80 mol, what new volume will result (at an unchanged temperature and pressure)?
Solution:
I'll use V1n 2 = V2n1
2 = V2n1(5.00 L) (1.80 mol) = (x) (0.965 mol)
x = 9.33 L (to three sig figs)
Example #2: A cylinder with a movable piston contains 2.00 g of helium, He, at room temperature. More helium was added to the cylinder and the volume was adjusted so that the gas pressure remained the same. How many grams of helium were added to the cylinder if the volume was changed from 2.00 L to 2.70 L? (The temperature was held constant.)
Solution:
1) Convert grams of He to moles:
2.00 g / 4.00 g/mol = 0.500 mol
2) Use Avogadro's Law:
V1 / n1 = V2 / n22.00 L / 0.500 mol = 2.70 L / x
x = 0.675 mol
3) Compute grams of He added:
0.675 mol − 0.500 mol = 0.175 mol(0.175 mol) (4.00 g/mol) = 0.7 grams of He added
Example #3: A balloon contains a certain mass of neon gas. The temperature is kept constant, and the same mass of argon gas is added to the balloon. What happens?
(a) The balloon doubles in volume.
(b) The volume of the balloon expands by more than two times.
(c) The volume of the balloon expands by less than two times.
(d) The balloon stays the same size but the pressure increases.
(e) None of the above.
Solution:
We can perform a calculation using Avogadro's Law:V1 / n1 = V2 / n2
Let's assign V1 to be 1 L and V2 will be our unknown.
Let us assign 1 mole for the amount of neon gas and assign it to be n1.
The mass of argon now added is exactly equal to the neon, but argon has a higher gram-atomic weight (molar mass) than neon. Therefore less than 1 mole of Ar will be added. Let us use 1.5 mol for the total moles in the balloon (which will be n2) after the Ar is added. (I picked 1.5 because neon weighs about 20 g/mol and argon weighs about 40 g/mol.)
1 / 1 = x / 1.5
x = 1.5
answer choice (c).
Example #4: A flexible container at an initial volume of 5.120 L contains 8.500 mol of gas. More gas is then added to the container until it reaches a final volume of 18.10 L. Assuming the pressure and temperature of the gas remain constant, calculate the number of moles of gas added to the container.
Solution:
V1 / n1 = V2 / n2| 5.120 L | 18.10 L | |
| –––––––– | = | –––––– |
| 8.500 mol | x |
x = 30.05 mol <--- total moles, not the moles added
30.05 − 8.500 = 21.55 mol (to four sig figs)
Notice the specification in the problem to determine moles of gas added. The Avogadro Law calculation gives you the total moles required for that volume, NOT the moles of gas added. That's why the subtraction is there.
Example #5: If 0.00810 mol neon gas at a particular temperature and pressure occupies a volume of 214 mL, what volume would 0.00684 mol neon gas occupy under the same conditions?
Solution:
1) Notice that the same conditions are the temperature and pressure. Holding those two constant means the volume and the number of moles will vary. The gas law that describes the volume-mole relationship is Avogadro's Law:
| V1 | V2 | |
| ––– | = | –––– |
| n1 | n2 |
2) Substituting values gives:
| 214 mL | V2 | |
| ––––––––– | = | –––––––––– |
| 0.00810 mol | 0.00684 mol |
3) Cross-multiply and divide for the answer:
V2 = 181 mL (to three sig figs)When I did the actual calculation for this answer, I used 684 and 810 when entering values into the calculator.
4) You may find this answer interesting:
Dividing PV1 = n1RT by PV2 = n2RT, we getV1/V2 = n1/n2
V2 = V1n2/n1
What Is Avogadro's Number
V2 = [(214 mL) (0.00684 mol)] / 0.00810 mol
V2 = 181 mL
In case you don't know, PV = nRT is called the Ideal Gas Law. You'll see it a bit later in your Gas Laws unit, if you haven't already.
Example #6: A flexible container at an initial volume of 6.13 L contains 7.51 mol of gas. More gas is then added to the container until it reaches a final volume of 13.5 L. Assuming the pressure and temperature of the gas remain constant, calculate the number of moles of gas added to the container.
Solution:
1) Let's start by rearranging the Ideal Gas Law (which you'll see a bit later or you can go review it right now):
PV = nRTV/n = RT / P
R is, of course, a constant.
2) T and P are constant, as stipulated in the problem. Therefore, we can write this:
k = RT / P
where k is some constant.
3) Therefore, this is true:
V/n = k
4) Given V and n at two different sets of conditions, we have:
V1 / n1 = k
V2 / n2 = k
5) Since k = k, we have this relation:
V1 / n1 = V2 / n2
6) Insert data and solve:
6.13 / 7.51 = 13.5 / n(6.13) (n) = (13.5) (7.51)
n = [(13.5) (7.51)] / 6.13
n = 16.54 mol (this is not the final answer)
7) Final step:
16.54 − 7.51 = 9.03 mol (this is the number of moles of gas that were added)
Example #7: A container with a volume of 25.47 L holds 1.050 mol of oxygen gas (O2) whose molar mass is 31.9988 g/mol. What is the volume if 7.210 g of oxygen gas is removed from the container, assuming the pressure and temperature remain constant?
Solution #1:
1) Initial mass of O2:
(1.050 mol) (31.9988 g/mol) = 33.59874 g
2) Final mass of O2:
33.59874 − 7.210 = 26.38874 g
3) Final moles of O2:
26.38874 g / 31.9988 g/mol = 0.824679 mol
4) Use Avogadro's Law:
V1 / n1 = V2 / n225.47 L / 1.050 mol = V2 / 0.824679 mol
V2 = 20.00 L
Solution #2:
1) Let's convert the mass of O2 removed to moles:
7.210 g / 31.9988 g/mol = 0.225321 mol
2) Subtract moles of O2 that got removed:
1.050 mol − 0.225321 mol = 0.824679 mol
3) Use Avogadro's Law as above.
Solution #3:
1) This solution depends on seeing that the mass ratio is the same as the mole ratio. Allow me to explain by using Avogadro's Law:
| V1 | V2 | |
| –––– | = | –––– |
| n1 | n2 |
2) Replace moles with mass divided by molar mass:
| V1 | V2 | |
| –––––––––– | = | –––––––––– |
| mass1 / MM | mass2 / MM |
3) Since the molar mass is of the same substance (oxygen in this case), they cancel out leaving us with this:
| V1 | V2 | |
| –––– | = | –––– |
| mass1 | mass2 |
4) Solve using the appropriate values
| 25.47 L | V2 | |
| –––––––– | = | –––––––– |
| 33.59874 g | 26.38874 g |
V2 = 20.00 L
Example #8: What volume (in L) will 5.5 g of oxygen gas occupy if 2.2 g of the oxygen gas occupies 3.0 L? (Under constant pressure and temperature.)
Solution:
1) State the ideal gas law:
| P1V1 | P2V2 | |
| ––––– | = | ––––– |
| n1T1 | n2T2 |
Note that it is the full version which includes the moles of gas. Usually a shortened version with the moles not present is used. Since grams are involved (which leads to moles), we choose to use the full version.
2) The problem states that P and T are constant:
| V1 | V2 | |
| ––– | = | ––– |
| n1 | n2 |
3) Cross-multiply and rearrange to isolate V2:
V2n1 = V1n2V2 = (V1) (n2 / n1)
4) moles = mass / molecular weight:
n = mass / mwV2 = (V1) [(mass2 / mw) / (mass1 / mw)]
5) mw is a constant (since they are both the molecular weight of oxygen), which means it can be canceled out:
V2 = (V1) (mass2 / mass1)
6) Solve:
V2 = (3.0 L) (5.5 g / 2.2 g)V2 = 7.5 L
Example #9: At a certain temperature and pressure, one mole of a diatomic H2 gas occupies a volume of 20 L. What would be the volume of one mole of H atoms under those same conditions?
Solution:
One mole of H2 molecules has 6.022 x 1023 H2 molecules.One mole of H atoms has 6.022 x 1023 H atoms.
The number of independent 'particles' in each sample is the same.
Therefore, the volumes occupied by the two samples are the same. The volume of the H atoms sample is 20 L.
By the way, I agree that one mole of H2 has twice as many atoms as one mole of H atoms. However, the atoms in H2 are bound up into one mole of molecules, which means that one molecule of H2 (with two atoms) counts as one independent 'particle' when considering gas behavior.
Example #10: A flexible container at an initial volume of 6.13 L contains 8.51 mol of gas. More gas is then added to the container until it reaches a final volume of 15.5 L. Assuming the pressure and temperature of the gas remain constant, calculate the number of moles of gas added to the container.
Solution:
1) State Avogadro's Law in problem-solving form:
| V1 | V2 | |
| ––– | = | –––– |
| n1 | n2 |
2) Substitute values into equation and solve:
| 6.13 L | 15.5 L | |
| ––––––– | = | –––––– |
| 8.51 mol | x |
x = 21.5 mol
3) Determine moles of gas added:
21.5 mol − 8.51 mol = 13.0 mol (when properly rounded off)
Bonus Example: A cylinder with a movable piston contains 2.00 g of helium, He, at room temperature. More helium was added to the cylinder and the volume was adjusted so that the gas pressure remained the same. How many grams of helium were added to the cylinder if the volume was changed from 2.00 L to 2.50 L? (The temperature was held constant.)
Solution:
1) The two variables are the volume and the amount of gas (temp and press are constant). The gas law that relates these two variables is Avogadro's Law:
| V1 | V2 | |
| ––– | = | –––– |
| n1 | n2 |
2) We convert the grams to moles:
2.00 g / 4.00 g/mol = 0.500 mol
3) Now, we use Avogadro's Law:
| 2.00 L | 2.50 L | |
| –––––––– | = | –––––– |
| 0.500 mol | x |
x = [(0.500 mol) (2.50 L)] / 2.00 L
x = 0.625 mol <--- this is the ending amount of moles, not the moles of gas added
4) This is the total moles to create the 2.50 L. We need to convert back to grams:
(4.00 g/mol) (0.125 mol) = 0.500 g <--- this is the amount added.Notice that I subtracted 0.500 mol from 0.625 mol and used 0.125 mol in the calculation. This is because I want the amount added, not the final ending amount.
| Boyle's Law | Combined Gas Law |
| Charles' Law | Ideal Gas Law |
| Gay-Lussac's Law | Dalton's Law |
| Diver's Law | Graham's Law |
| No Name Law | Return to KMT & Gas Laws Menu |
